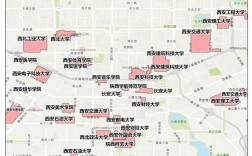
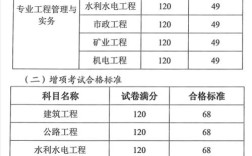
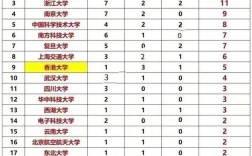
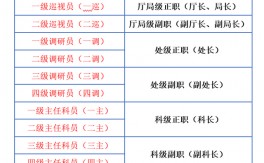
arctanx的不定積分
函數 \(\arctan(x)\) 的不定積分可以通過積分的部分分式方法來求解。不定積分 \(\int \arctan(x) \, dx\) 的結果是:
\[
\int \arctan(x) \, dx = x \arctan(x) - \frac{1}{2} \ln(1+x^2) + C
\]
其中 \(C\) 是積分常數。這個結果可以通過使用積分技巧和一些代數操作得到。簡單來說,這個積分涉及到對反正切函數的積分,這通常需要一些積分技巧來解決。

∫arctanxdx的詳解
積分 \(\int \arctan x \, dx\) 可以通過部分積分法來求解。部分積分法是積分學中的一種方法,用于計算兩個函數乘積的積分,公式如下:
\[
\int u \, dv = uv - \int v \, du
\]
對于 \(\int \arctan x \, dx\),我們可以選擇 \(u = \arctan x\) 和 \(dv = dx\)。接下來,我們需要計算 \(du\) 和 \(v\)。
1. 計算 \(du\):
\[
u = \arctan x \implies du = \frac{1}{1+x^2} \, dx
\]
2. 計算 \(v\):
\[
dv = dx \implies v = x
\]
現在,我們可以應用部分積分公式:
\[
\int \arctan x \, dx = x \arctan x - \int \frac{x}{1+x^2} \, dx
\]
接下來,我們需要計算 \(\int \frac{x}{1+x^2} \, dx\)。這個積分可以通過長除法或者識別它是一個簡單的有理函數來解決。這個積分的結果是:
\[
\int \frac{x}{1+x^2} \, dx = \frac{1}{2} \ln |1+x^2| + C
\]
其中 \(C\) 是積分常數。
將這個結果代入部分積分的公式中,我們得到:
\[
\int \arctan x \, dx = x \arctan x - \frac{1}{2} \ln |1+x^2| + C
\]
這就是 \(\int \arctan x \, dx\) 的積分結果。
不定積分表
不定積分是微積分中的一個重要概念,它與導數的概念相對應。不定積分表是包含了一些基本函數與其對應不定積分的表格,用于快速查找積分結果。以下是一些常見的函數及其不定積分:
1. \( \int x^n dx = \frac{1}{n+1}x^{n+1} + C \) (其中 \( n \neq -1 \))
2. \( \int \frac{1}{x} dx = \ln|x| + C \)
3. \( \int e^x dx = e^x + C \)
4. \( \int a^x dx = \frac{1}{\ln a}a^x + C \) (其中 \( a > 0 \) 且 \( a \neq 1 \))
5. \( \int \sin x \, dx = -\cos x + C \)
6. \( \int \cos x \, dx = \sin x + C \)
7. \( \int \tan x \, dx = -\ln|\cos x| + C \)
8. \( \int \cot x \, dx = \ln|\sin x| + C \)
9. \( \int \sec x \, dx = \ln|\sec x + \tan x| + C \)
10. \( \int \csc x \, dx = -\ln|\csc x + \cot x| + C \)
11. \( \int \frac{1}{1+x^2} dx = \arctan x + C \)
12. \( \int \frac{1}{\sqrt{1-x^2}} dx = \arcsin x + C \)
13. \( \int \frac{1}{x\sqrt{x^2-1}} dx = \frac{1}{2}\ln\left|\frac{x-\sqrt{x^2-1}}{x+\sqrt{x^2-1}}\right| + C \) (\( x > 1 \))
14. \( \int \frac{1}{x^2-a^2} dx = \frac{1}{2a}\ln\left|\frac{x-a}{x+a}\right| + C \) (\( a > 0 \))
15. \( \int \frac{1}{x^2+a^2} dx = \frac{1}{a}\arctan\left(\frac{x}{a}\right) + C \) (\( a > 0 \))
16. \( \int \frac{1}{\sqrt{x^2+a^2}} dx = \ln|x+\sqrt{x^2+a^2}| + C \) (\( a > 0 \))
17. \( \int \frac{1}{\sqrt{x^2-a^2}} dx = \ln|x+\sqrt{x^2-a^2}| + C \) (\( x > a \))
18. \( \int \sin ax \, dx = -\frac{1}{a}\cos ax + C \)
19. \( \int \cos ax \, dx = \frac{1}{a}\sin ax + C \)
20. \( \int e^{ax} dx = \frac{1}{a}e^{ax} + C \) (\( a \neq 0 \))
這些是一些基本的不定積分公式,對于更復雜的函數,可能需要使用積分技巧如部分積分、換元積分法等來求解。

 微信掃一掃打賞
微信掃一掃打賞